
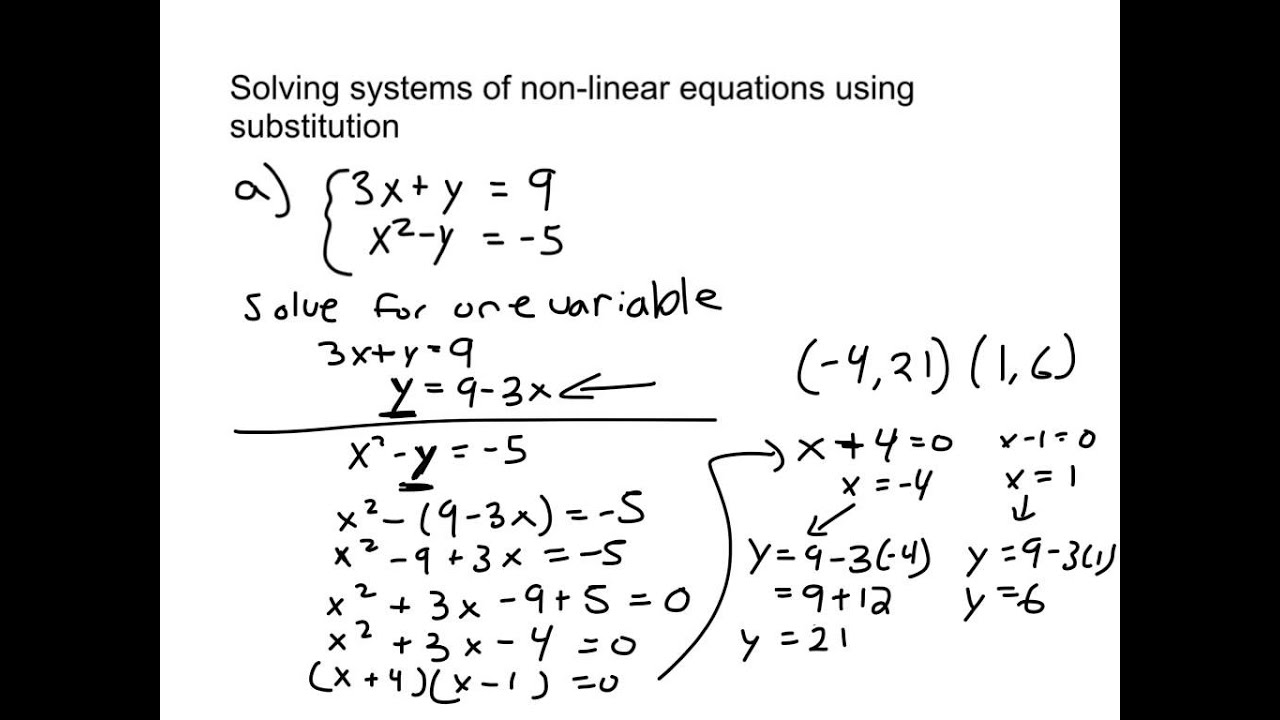
Then, we use this rearranged equation and substitute it for every time that variable appears in the other equations.

The first method that students are taught, and the most universal method, works by choosing one of the equations, picking one of the variables in it, and making that variable the subject of that equation. Let's briefly describe a few of the most common methods. There are many different ways to solve a system of linear equations. For example, the equation -2x + 14y - 0.3z = 0 is linear, but 10x - 7y + z² = 1 isn't. This applies to all the variables in an equation. They can, however, be multiplied by any number, just as we had the 3 in our 3x = 30 equation. This means that, for instance, they are not squared x² as in quadratic equations, or the denominator of a fraction, or under a square root. " But what the heck does linear mean?" We say that an equation is linear if its variables (be they x's or coconuts) are to the first power. In essence, " what is the solution to the system of equations." is the same as " give me the value of an apple (or x) that satisfies." To be honest, we know that most scientists would love to use bananas instead of x's, but they're just insecure about their drawing skills. In our case, we know that three apples equal to 30, but the apple is simply a variable, like x, as we don't know the value of it. It denotes a number or element that we don't know the value of, but that we do know something about.
The x that appeared above is what we call a variable.


 0 kommentar(er)
0 kommentar(er)
